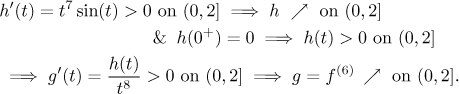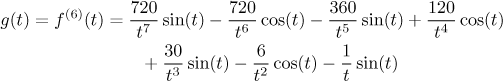
Here we will show that the function

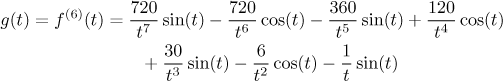
is increasing on the interval M = (0,2]. First off, note that we cannot see any monotonicity right away. Since cos(t) is decreasing and t^k is increasing on M, the ratio cos(t)/t^k is decreasing there. Now such terms are both with plus and with minus in our g and we know that difference of two decreasing functions can be anything, decreasing or increasing or not monotone at all; to top it all we have the terms with sine there, which shows that this approach leads nowhere. There seems to be no other algebraic approach, so we try it the traditional way, via derivative.

Solving g′ = 0 is impossible algebraically, the only chance is to use computer to find at least approximate solutions numerically, but even that would be rather tricky and difficult. Therefore we need to resort to non-standard tactics and tricks. The key observation here is that we do not really need to solve that equation; we just need to show that g′ > 0 on M. We start by rewriting this derivative using comon denominator.

Since the denominator is positive, it is enough to prove that the numerator h is also positive on M. Again, doing it somehow algebraically seems impossible, so we do it by investigating how h goes; that is, we apply the standard monotonicity approach. First we find its derivative and here a little miracle happens. Each term is differentiated using the product rule and thus produces two terms. By a remarkable coincidence, neighboring terms cancel out and the whole long expression (15 terms in all) collapses on itself. Check that

h′(t) = t⁷sin(t).

This is clearly positive on M, so h itself is increasing there. Thus the range of values it can reach is given by values at the endpoints.

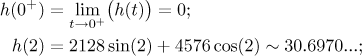
We see that on M the values of h are between 0 and 31, so in particular h must be positive and the proof is finished.

Just to show how all pieces of this puzzle fit together, we briefly reconstruct the chain of argument here.