Hyperbolic functions

These functions are surprisingly similar to trigonometric functions, although they do not have anything to do with triangles. The similarity follows from the similarity of definitions. At the end of this section we mention another reason why trigonometric and hyperbolic functions might be close.

Definition.
The hyperbolic sine and hyperbolic cosine are defined by

The hyperbolic tangent and hyperbolic cotangent are defined by

The hyperbolic sine. The domain:

D(sinh) = ℝ.

The graph:

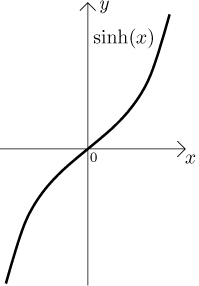
The function is continuous on its domain, unbounded, and symmetric, namely odd, since we have sinh(−x) = −sinh(x).

There is one zero point, namely x = 0, which is also a point of inflection. There are no local extrema, limits at endpoints of the domain are

The derivative:

[sinh(x)]′ = cosh(x).

The hyperbolic cosine. The domain:

D(cosh) = ℝ.

The graph:

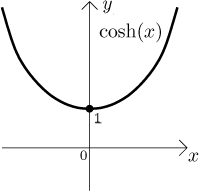
The function is continuous on its domain, bounded from below, and symmetric, namely even, since we have cosh(−x) = cosh(x).

There is no zero point, but a local minimum at x = 0, the function is always concave up. Limits at endpoints of the domain are

The derivative:

[cosh(x)]′ = sinh(x).

The hyperbolic tangent. The domain:

D(tanh) = ℝ.

The graph:

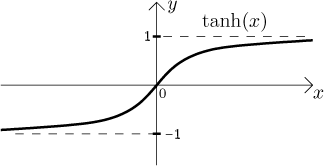
The function is continuous on its domain, bounded, and symmetric, namely odd, since we have tanh(−x) = −tanh(x).

There is a zero point, namely x = 0, which is also a point of inflection. There are no local extrema, limits at endpoints of the domain are

The derivative:

[tanh(x)]′ = 1/cosh²(x).

The hyperbolic cotangent. The domain:

D(coth) = ℝ − {0} = (−∞,0) ∪ (0,∞).

The graph:

The function is continuous on its domain, unbounded, and symmetric, namely odd, since we have coth(−x) = −coth(x).

There is no zero point and no point of inflection, there are no local extrema. Limits at endpoints of the domain are

The derivative:

[cotgh(x)]′ = −1/sinh²(x).

Note that we often write sinhⁿ(x) instead of the correct [sinh(x)]ⁿ, similarly for the other hyperbolic functions.

The following identities are very similar to trig identities, but they are tricky, since once in a while a sign is the other way around, which can mislead an unwary student.

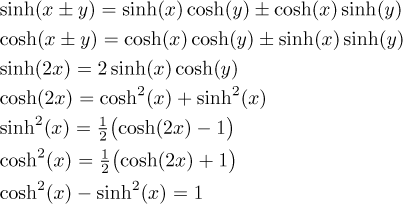
The identities for hyperbolic tangent and cotangent are also similar.

Here the situation is much better than with trig functions. Apart from the hyperbolic cosine, all other hyperbolic functions are 1-1 and therefore they have inverses. To get the inverse of cosh(x), we restrict it to the interval [0,∞). The inverse functions are called argument of hyperbolic sine, denoted argsinh(x), argument of hyperbolic cosine, denoted argcosh(x), argument of hyperbolic tangent, denoted argtanh(x), and argument of hyperbolic cotangent, denoted argcoth(x). Their graphs are

Basic properties:

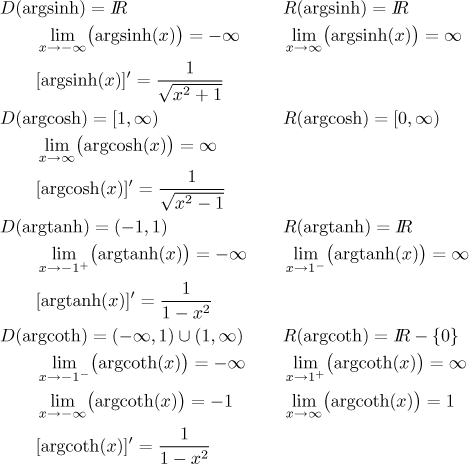
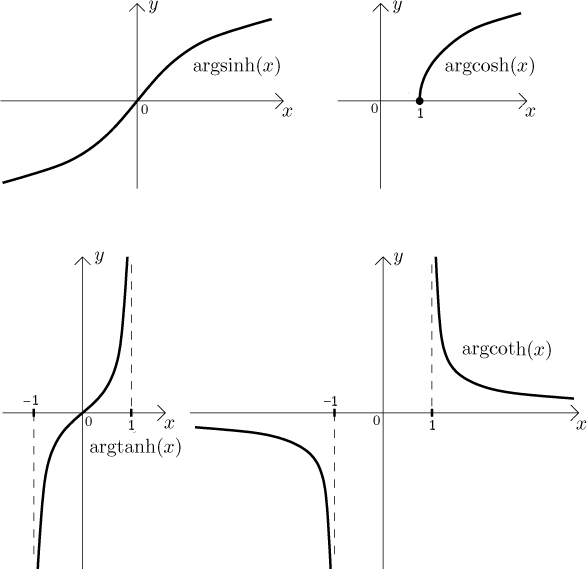
Now we come to another advantage of hyperbolic functions over trigonometric functions. We actually have "nice" formulas for the inverses:

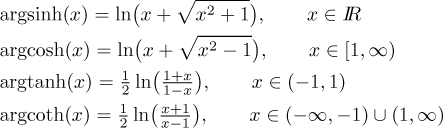
Note: The inverse functions are also sometimes called "area hyperbolic functions". There are two alternative notations, instead of argsinh(x) some would write arcsinh(x) or sinh⁻¹(x). The first notation is probably inspired by inverse trig functions, the second one is unfortunately quite prevalent, but it is extremely misleading. The reason is that many students see an admitedly existing similarity between sinh⁻¹(x) and sinh²(x), so they think that sinh⁻¹(x) is actually 1/sinh(x). We talked about some justification for this misleading notation when we introduced inverse functions in Theory - Real functions. Still it is very unfortunate, especially since there is a perfectly adequate arg-notation that we introduced above. We will stick to it here in Math Tutor.

Note on parametric curves

One interesting property of trig functions is that they provide a nice description of a circle. Indeed, the circle of radius r centred at the origin (given by x² + y² = r² in Cartesian coordinates) is given by the parametric equations x = r⋅cos(t), y = r⋅sin(t).

What happens if we replace these functions with their hyperbolic cousins? The equations x = r⋅cosh(t), y = r⋅sinh(t) describe exactly the right branch of the rectangular hyperbola x² − y² = r².

Absolute value
Back to Theory - Elementary functions