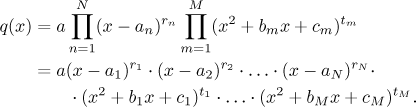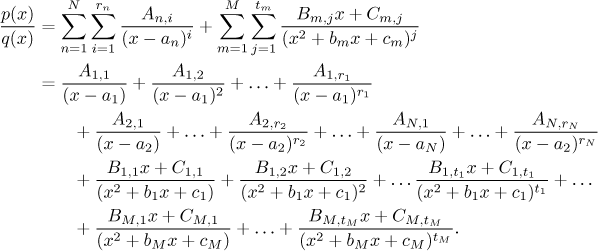This method is used when working with rational functions, that is, ratios of polynomials. Obviously, we will use it for integration here. Here it comes.
Theorem (partial fractions decomposition)
Let p and q be real polynomials, let the degree of p be less than the degree of q. Assume that q can be factored as follows:
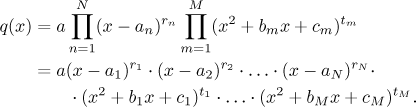
where the factors are mutually distinct and the quadratic terms are irreducible (without real roots). Then we have the following decomposition:
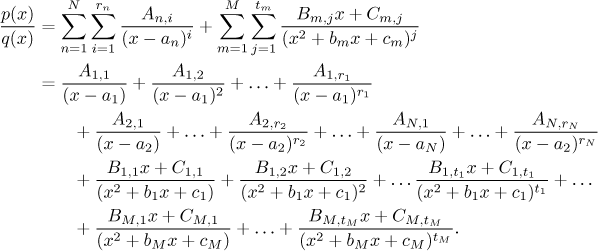
and all the constants A, B a C are uniquely determined by the denominator of the corresponding partial fraction.
What does it tell us? Imagine that we have two polynomials,
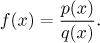
We may assume that this rational function is "proper", that is, the polynomial in the numerator has a lower degree than the one in the denominator. This theorem says that a problem that features perhaps even a very complicated ratio can be reduced to ratios that are markedly simpler, in fact they are chosen from two basic types, so-called partial fractions.
For every linear factor
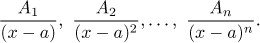
For every irreducible quadratic factor
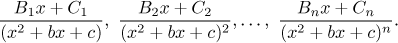
As an example convince yourself that the following equality is true.
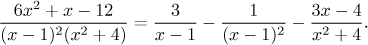
The theorem also says that the decomposition can be done in just one way. On the other hand, it does not tell us how to find the coefficients in the partial fractions, which is not so surprising, there are no universal formulas for them. There are several methods for finding these numbers and we will discuss it in the section on rational functions in Methods Survey - Methods of integration.
