Problem: Determine the constants a,b for which the following function is continuous.

Solution: Since both a^x and the expression in the third alternative are continuous functions on their domais, it follows that the given function f is also continuous on open sets where it is given by these expressions. Thus we have continuity at all points apart from 2. We see that this question is really about continuity at 2. What is needed there? We need both one-sided limits to exist, converge and be equal to f (2) = 8.

We start with the limit from the left, to the left from 2 the function is given by a^x.

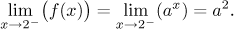
For continuity we need f (2^-) = 8, that is, a² = 8. This equation has two solutions, plus and minus root of 8, but since a is supposed to be the base of an exponential, it cannot be negative. Thus we set a to be the positive root of 8 to get continuity from the left.

Now we address continuity from the right, to the right from 2 the function is given by the fraction.

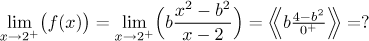
If we want to have any chance of convergence, we need to neutralize the zero in the denominator. One possibility is to put b = 0, but then the whole expression is identically zero and therefore also f (2⁺) = 0, so there si no chance of making this into 8. Therefore 0 is not the right choice for b. The other possibility is to make the numerator zero in the limit, which means that b² should be 4. There are two possibilities, b can be −2 or 2, and we check whether any of them makes the limit equal to 8. Note that these two values of b are the only hopes for making the function continuous at 2 and if none of them work out, then the function simply cannot be made continuous at 2.

First we try b = −2.

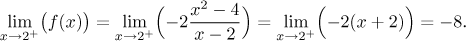
This is no good. We try b = 2.

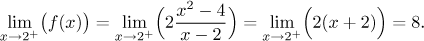
We got lucky, this is the right value for b.

Conclusion: The function f is continuous for the values

Next problem
Back to Solved Problems - Real functions