Problem: Determine whether the following series converges.
![]()
Solution: The terms of this series are positive, so all the nice tests are available. Given the form of the terms, the most obvious choice is the Root test.
![]()
Unfortunately,

Yes, it was ugly and pointless.
Since we do not know how to integrate the given expression, the Integral test is also out. How about comparison? We see one comparison right away, an obvious inequality allows us to drop those "1".
![]()
The test series on the right is divergent and larger, so this is exactly the situation when the Comparison test allows no conclusion.
How about the Limit comparison test that allows implication in both directions? There is a little problem here. If it was just the fraction, one might argue that adding and subtracting "1" does not make any difference for large k and instead of inequality above we may claim that
![]()
Unfortunately, intuitive reasoning works only for fractions, not for fractions raised to power k. Why is it so? Ignoring those "1" makes only little difference compared to dominant parts in the fraction, but when "something plus little" is raised to infinity, that "little" may start playing a big role. Still, it is worth trying. Is it true that ak is about 1?
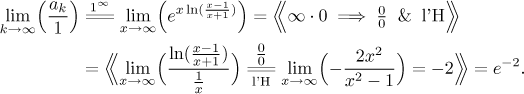
We see that our guess was wrong indeed, the terms do not look like 1 for large k. However, this is not the end of limit comparison, since this test does not require almost equality, just rough similarity. That is, the formal requirement in the Limit comparison test is exactly what we obtained, that the limit comes up positive. Thus the Limit comparison test in fact works, it shows that
![]()
and we know that the series on the right diverges. Consequently, the given series diverges.
Actually, the above calculation shows that the limit of ak is not zero, so by the necessary condition of convergence the given series diverges. This is probably the shortest solution, just find the limit of ak and since it is not zero, the series is divergent.
Why didn't we start with this test right away? Because in 99 cases out of 100 it ends up non-conclusive, so if we do not see it at the first glance (our case), then it is usually not worth wasting our time on it, it is better to try something else. In this particular problem it did not work out.